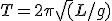The Pendulum Swings

Sometimes I’m just reading, minding my own business, when the oddest things smack me squarely in the forehead. For instance:

As believers in faith and ritual over science, perhaps it’s not surprising that they [Evangelical Christians, as it turns out] failed to heed the basic laws of physics.

Most people understand that when a pendulum is pushed too far in one direction, it will eventually, inexorably swing back just as far to the opposite side. This is the natural order of things, and it tends to apply across the board — even to that bulwark of chaos theory, politics.

[Chez Pazienza, "Losing Their Religion", Huffington Post, 30 January 2008]

Whatever is this person talking about and where did s/he get the crazy notions about “the basic laws of physics” on display in these few sentences? (It seems about as nonsensical to me as people who use “literally” to mean “really, really metaphorically”.)

Based on the laws of physics, I believe that a pendulum is a physical object that swings back and forth, often used to keep time. I also believe that if it’s pushed far enough in one direction is will eventually break or, at the very least, enter a non-linear mode of oscillations. In my book, it is in the nature of pendula, even when swung a little in one direction, to swing in the other direction, and then back again in the original direction.

It is this oscillatory nature of the pendulum that is referred to in the metaphorical pendulum of politics and public opinion. Perhaps our author is thinking of a spring that, when squeezed, or stretched, in one direction will spring back just as far in the opposite direction?

As for politics being the bulwark of chaos theory — WTF? Someday, perhaps when we have more time, we’ll talk about some interesting history and results in chaos studies, but I don’t think politics will get mentioned, alas.

A pendulum is a fascinating thing, of course. Its use in clocks as a timing governor^* is traced to Galileo’s observation that the period of oscillation depends only on the length of the pendulum and not on the amplitude of its swing. The period (“T”) depends only on the length (“L”) of the pendulum and the acceleration due to gravity (“g”–a constant number):

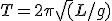
Now, this is really an approximation with some assumptions like a) the pendulum has all its weight at the swinging end; and b) the amplitude of the swing isn’t too big. But it’s really a very good approximation, good enough for very precise horological instruments.

This equation tells us a couple of interesting things. One is that, because of the square-root sign over the length, if you want to double (multiply by 2) the period of a pendulum you must increase its length by 4; likewise, for half the period make the length one-fourth the original.

This also tells us that tall-case clocks tend to be much the same size. Generally speaking, they are constructed to house a pendulum with a two-second period, i.e., a pendulum that takes precisely one second to swing either way, or one second per tick, one second per tock. The length of such a pendulum is very nearly 1 meter.

At our house we also have a mantel clock that is, not surprisingly, a little under 12 inches tall because it has a pendulum with a period of 1 second, i.e., one second for a complete back-and-forth swing; such a pendulum has a length of about 0.25 meters, or one-quarter the tall-case clock’s pendulum.

Many tall-case clocks that I’ve seen have a pendulum whose rod is actually made from a flat array of a number of small rods, usually in alternating colors. This is a merely decorative vestige of the “gridiron pendulum” invented by master horologist John Harrison in 1720. The pendulum is constructed of two types of metal arranged so that the thermal expansion of one type of metal is compensated for by the thermal expansion of the other. (It’s easiest to look at an illustration, which is discussed here.)
———-
^* The pendulum, coupled with an escapement mechanism, is what allows a pendulum clock to tick off uniform intervals in time.

The “Cells” in Cell Phones

Isaac & I returned home yesterday, flying from Kansas City (central standard time) to Washington, DC (eastern standard time). As we arrived at the gate in DC, I overheard this conversational exchange from the seats in front of mine:

Mother: Oh, look! My cell phone has changed back to eastern time.
Teen-age son: That’s because they work with satellites, and they know where you are.

I was a bit surprised that, contrary to common belief, young people still don’t know everything. There is at least one surprising misconception in the son’s mind that I should have cleared up on the spot, but I didn’t. Y’all are so lucky.

Modern mobile phones, known also as cell phones, do not communicate through satellites, and they never have. There have existed satellite telephones that do, but they’re a much different beast, not to mention much larger and much heavier.

Cell phones communicate with cell towers–or, more generally, cell sites, since not all cellular antennae are on towers; many are hidden on building tops, for instance. If the cell sites are visible they are easily recognizable, most often triangular structures with vertical “bars” on each face of the triangle. Each of the “bars” is actually an antenna for transmitting to mobile phones or else receiving signals from them. The antennae are used in pairs so that they send and receive signals directionally.

The whole idea of “cells” was originally the way to provide coverage over a wide area without requiring a large amount of power in the handset, and also as a way to use restricted amounts of radio-frequency bandwidth efficiently and provide for a number of users.

In some area over which the cellular provider wants coverage, the area is divided into hexagonal “cells” that cover the area. (Look at a bathroom floor sometime that has 6-sided tiles and you will see that the area can be completely covered without gaps.) At the center of each cell is a cell site. The cell site has the three-sided shape so that it can hear in all directions. A cell site is responsible for all the cellular phones in its cell.

The imagined boundaries of the cells overlap a bit, so each cell actually operates on a slightly different frequency^# from all of its neighbors. Because of that, cell sites over a wider area can reuse frequencies, but there is the added technical challenge of tracking a particular cell phone between the ranges of neighboring cell sites and switching an active conversation from being routed through one cell site to being routed to another without dropping the call. That process is call “handover”. When a particular phone switches cells it also shifts the frequency that it uses for the radio link by a small amount.

The size of each cell varies depending on terrain and obstructions and such things, but in denser areas cell sites will be about 5 to 8 miles apart, so that’s the furthest that you cell phone usually has to transmit its signal, which is something it can manage to do with the relatively tiny batteries that it carries.

Cell sites do not continuously track a cell phone unless the phone is engaged in a conversation. If your phone has been off, it will always talk with the nearest cell site when you turn it back on and the phone network takes note of your position. Occasionally every cell sites will query phones with broadcast messages; the phones respond, and that way your cellular network can quickly find which cell site to use to contact your phone when you are receiving a call. The cell sites also broadcast timing signals, which is how cell phones always seem to know the right time. Note this: the cell site doesn’t have to know where your phone is to get the time correct, instead your phone simply takes the local time of the cell site that it can hear.

Now, one last note about cell phones talking through satellites. Most communications satellites are in geostationary orbits, which means they are in orbits where they appear stationary in the sky. This is where you will find the satellites that broadcast satellite radio and television, too.

Anyway, to be in a geosynchronous orbit requires that the satellite be at an altitude of about 22,000 miles. That’s a long way compared to the 8-mile distance to the nearest cell site. In fact, it’s about 2,750 times as far away. Other things being equal^* that means that the cell phone would require 7.6 million times the power for its signal to reach the satellite. As you might guess, the would require a much bigger battery than your cell phone has in it.

By the way, before I finish, I have to chastise the FCC for poor technical writing. I was looking around for a few details about cell-phone networks and I found this page of “Cell Phones FAQs” from “The FCC Kids [sic] Zone”. You may wish to look at the answer for “How Does a Cell Phone Work” and count how many errors and imprecise statements you can find in one paragraph. (For extra credit, read the other answers if you can stand it.) There are many irritants, but I could start with the absurdity of using the copper-wire based, home phone system as a conceptual basis, since kids don’t use that archaic communication system anymore as a conceptual referent.

There there’s this statement:

A cell phone turns your voice into a special type of electricity and sends it over the air to a nearby cell tower; the tower sends your voice to the person you are calling.

Calling propagating electromagnetic waves a “special type of electricity” is incorrect and unnecessary, an egregious error. Saying the tower “sends your voice” is no better. Despite what this FCC author seemed to think, it’s entirely possible not to go into pages of detail about the time-slice multiplexing and analog-signal digitization (most cell networks these days are digital) used to “send your voice” over the network and still get it right without the stupid and inaccurate “send your voice” gambit.

These are just the type of gratuitous and imprecise over-simplifications about science and technology that drive me into a frenzy and that I have vowed that Ars Hermeneutica will combat. If any of my four regular readers happen to know someone at the FCC, have them get in touch and we can straighten out these things before any more bad ideas get into kids’ (note the apostrophe) heads.
———-
^# Cell phones also use a different frequency to transmit from the frequency they use for receive, but that’s a needless conceptual complication at this stage.

^* There are details, naturally. The 7.6 million number is the square of 2,750, because radiated electromagnetic power diminishes as the square of the distance. However, satellite communications is possible with these geosynchronous satellites because their receivers have much higher gain (i.e., can hear much weaker signals) than terrestrial cell sites. They also are much too far away to be able to break an urban region up into cells and distinguish calls from different cells, let alone transmit in different cells, but that’s a whole other story.

Approaching Mars

Before I even get that ridiculous e-mail about how Mars will soon look as big as the moon because of a close approach by the Earth, here’s a note from NASA:

August 21, 2007: By the time you finish reading this sentence, you’ll be 25 miles closer to the planet Mars.

Earth and Mars are converging, and right now the distance between the two planets is shrinking at a rate of 22,000 mph–or about 25 miles per sentence. Ultimately, this will lead to a close approach in late December 2007 when Mars will outshine every star in the night sky. Of a similar encounter in the 19th century, astronomer Percival Lowell wrote the following: “[Mars] blazes forth against the dark background of space with a splendor that outshines Sirius and rivals the giant Jupiter himself.”

Contrary to rumor, though, Mars is never going to outshine the Moon.

There is an email circulating the internet—called the “Mars Hoax” or the “Two Moons email”—claiming that Mars will soon swell as large as the full Moon, and the two will hang together side by side on the night of Aug. 27th. “Mars will be spectacular,” it states. “No one alive today will ever see this again.”

No one will see it, because it won’t happen.

It is true that Earth and Mars are converging–you’re now 300 miles closer–but even at closest approach the two planets are separated by a gulf of tens of millions of miles. From such a distance, Mars looks like a star, an intense yet tiny pinprick of light, never a full Moon.

[excerpt from Dr. Tony Phillips, "Hurtling Towards Mars", Science @ NASA, 21 August 2007.]

I rather like the poetry of their special-purpose units for velocity: miles / (sentence read).

Reason vs. Faith, Again

This week Bob Park (What’s New for 1 June 2007) revisits presidential candidate Sam Brownback’s positive response when asked during a debate whether he was one who did not “believe” in evolution:

BELIEFS: BROWNBACK DEFENDS SCIENTIFIC ILLITERACY BY EXAMPLE.
A month ago at the Republican Presidential debate, there was a show of hands of those who don’t believe in evolution. One who raised his hand, Sam Brownback, was moved to explain why in yesterday’s New York Times: “I believe wholeheartedly that there cannot be any contradiction between faith and reason.” Which faith does he have in mind? Different faiths are often at war with each other, but no wars are fought over science. Science relies on Nature as the sole arbiter. There was much more, all in the language of the intelligent design movement, including the substitution of “materialism” for “naturalism.”

The op-ed in question is “What I Think About Evolution” (Sam Brownback, New York Times, 31 May 2007). In it he, apparently, tries to soften his position and find a way to say that he doesn’t not believe in evolution, mostly by trying to deny most of what evolution is and is all about, and then claiming that he doesn’t not believe in that. It’s not a successful tactic.

In matters of conflict between science and theology, there is a famous aphorism of the late John-Paul II: “Truth cannot contradict truth”, which is to say that if there is an apparent conflict between theological truth and scientific truth, it must be apparent only and due to incomplete understanding, because “truth cannot contradict truth”. Not so long ago I wrote an essay on the matter (“Evolution and the Vatican“), in which I ended up tracing the “truth cannot contradict truth” idea back to Pope Leo XIII, and then following forward papal writings and attitudes about evolution. In the context of mature Catholic theology it makes clear sense. Once again, it reminds me of my feeling that a mature theology like that of the Catholic church makes what passes for fundamentalist theology seem juvenile and exceedingly simple-minded by comparison.

Unfortunately, Mr. Brownback misunderstands and perverts the deep significance of “truth cannot contradict truth” — quite knowingly, I suspect — by offering in his op-ed “clarification” this updated fundamentalist version:

The heart of the issue is that we cannot drive a wedge between faith and reason. I believe wholeheartedly that there cannot be any contradiction between the two.

In other words: “faith cannot contradict reason”. Or, I suspect, he’d prefer “reason cannot contradict faith”, because he goes on to say that “Faith seeks to purify reason…”, which does not indicate a comparison of equals. He seems to assert that reason and faith are equally reliable except when there’s a contradiction, then faith wins — of course.

“Faith” is not interchangeable with a concept like “theological truth”. Faith, claimed as a revelation by the faithful, has virtually no connection to theological debate — debate is not necessary — and no connection to the use of reason which, in the context of a mature theology, is a God-given faculty provided to assist in the discovery of “truth”. “Faith” is a personal matter, but hardly the foundation of doctrine or theology.

Is this a naive misinterpretation of the John-Paul II aphorism, or a willful bending to suit Brownback’s own purposes? Either one is deplorable and neither does much to bolster Brownback’s claim that he doesn’t reject evolution, well, not reject entirely. In my opinion Brownback has only dug his hole deeper, but I’m sure his supporters will have faith that it brings him closer to heaven.

Exponential Growth

Here’s a quick question with a pedagogical purpose. Would you buy a battery from this man?

“The energy capacity of batteries is increasing 5 percent to 8 percent annually, but demand is increasing exponentially,” Mr. Cooper[, vice president for business development of PolyFuel Inc., a company working on battery technology,] said.

[Damon Darlin and Barnaby J. Feder, "Need for Battery Power Runs Into Basic Hurdles of Science", New York Times, 16 August 2006.]

Forget basic hurdles of science, the basic hurdle here would seem to be an executive in a technical industry who doesn’t understand what exponential growth is.

In short: growth of something that is proportional to the current size of that thing is exponential growth. Thus, demand for batteries that grows 5% to 8% annually — i.e., 0.05 to 0.08 times current demand — is exponential growth.

The constant that governs how fast something grows exponentially is the “growth rate”. Small growth rate = slow growth; large growth rate = fast growth. In symbols, an exponential function of time, t, is

f(t) = A × e^st

where A is a constant amplitude and s is the growth rate. If s is relatively large, f(t) changes values rapidly; is s is very small, f(t) changes values slowly. If s happens to be a negative number, f(t) disappears over time, quickly or slowly depending on the size of s. The letter ‘e’ represents the base of natural logarithms. Why it shows up in the exponential function takes some explanation; for now, just think of it as a constant number nearly equal to 2.17 and don’t lose any sleep over it.^*

Many people think “exponential growth” means “grows really, really quickly”, but this is a misconception. It is true that power-law growth is generally faster than algebraic growth (for instance, multiplying a number over and over again by some number, say, 47) all other things being equal, but any particular exponential function will grow slowly or quickly depending on its growth rate. Think of a $0.15 deposit in a bank account that pays compound interest; the account grows exponentially but it’s going to be awhile before you’re a millionaire.

So please, please can we stop saying things like “Wow! That growth is so exponential! It’s huge!”

And if I were you, I don’t think I’d buy a battery from Mr. Cooper, either.
———-
^* In fact, ‘e’ is irrational (not expressible as the fraction of two integers, or whole numbers) and transcendental (not the solution to an algebraic equation, which is to say a polynomial with rational coefficients and integer powers). But that’s a lot of other story that we needn’t go into right now.

Don’t Need no Science

Is Bob Park’s What’s New for 11 May 2007, this quick summary of the Republican presidential-candidate field, demonstrating that science is not a conservative, traditional-family value and that Ars Hermeneutica has its work cut out for it:

BELIEFS: SCIENTIFIC ILLITERACY REACHES CLEAR TO THE TOP.
Last week at the Republican presidential debate, moderator Chris Matthews asked whether any of the wannabes did not believe in evolution. Sam Brownback, Mike Huckabee and Tom Tancredo raised their hands. John McCain waffled: “I believe in evolution, “he said, “but I also believe when I hike the Grand Canyon that the hand of God is there also.” The Sunday Washington Post pointed out that they weren’t that far from mainstream. In an ABC poll a year ago, 61% thought Genesis is literally true.

Exponential Dracula

Herewith, from the popular press, an excellent example of geometric (exponential) growth and its implications:

WASHINGTON (AP) — It may be the season for vampires, ghosts and zombies. Just remember, they’re not real, warns physicist Costas Efthimiou.
[...]
Efthimiou takes out the calculator to prove that if a vampire sucked one person’s blood each month [only 1 meal a month!] — turning each victim into an equally hungry vampire [exponential growth!] — after a couple of years there would be no people left, just vampires. He started his calculations with just one vampire and 537 million humans on January 1, 1600 and shows that the human population would be down to zero by July 1602.

[Associated Press, "Count Dracula not in the numbers, physicist says",^* cnn.com, 27 October 2006.]
———-
^* Evidently the headline writer was tired; a more obvious choice: “Count Numbers, not Dracula”.

Mystical Time

Not to harp on the innumeracy thing (although — plug time! — it is part of the mission of Ars Hermeneutica), but I’m a little irritated.

You see, I keep seeing people for the last few days pointing out, in e-mail and on their blogs^*, that on 5 April something unusual is going to happen. Namely, the time and date at one moment in the wee hourse will be

01:02:03 04/05/06

“This will never happen again!” is trumpeted along with the observation.

Of course, this is incorrect for two contradictory reasons: 1) it isn’t really happening in the first place; and 2) it will happen again.

Dispensing with #2 first, a moment’s reflection quickly shows that because only the last two digits of the year are used in the mystical rendering, this “reading” will happen precisely every 100 years, give or take depending on leap seconds or other adjustments; we could say it will happen exactly every 100 nominal years.

Now, for #1. It should come as no surprise that I am always troubled by mystical malarky like this, but I find it more troubling when it is so arbitrary, depending as it does only on accidents of the way we count time and keep track of days.

For most Europeans, the revelation won’t make any sense because they tend to write the day number before the month, 05/04/06, so clocks won’t get all mystical for them until 4 May. But should the mystical power depend so critically on using only two digits for the date? Oh dear, but 04/05/2006^# just doesn’t do it, does it? All this without even mentioning the different calendars and years observed by different cultures (China, say, or Orthodox Christians or Jews). Also not to mention that it depends on the fact that we divide the day up into 24 hours, and each hour in 60 minutes of 60 seconds each — like that’s natural and deeply meaningful!

I am not, however, totally immune to these accidental coincidences of digits. For instance, I find it useful — as a mnemonic — that Ars Hermeneutica’s incorporation was recorded in the Maryland Department of State at 11:11 on 11 November. Surely that must mean something!

———-
^* For example, Shakespeare’s Sis mentions it here.

^# Update added barely one hour before the mystical event here in the Central-Daylight-Time timezone: I just realized with some excitement that if only we more generally used a 24-hour clock and wrote our dates euro-style, we could look forward, in just a couple of months, to 20:06 20/06/2006, which is guaranteed never to happen again with our current Gregorian calendar.

All In Perspective

From a report about Exxon’s latest record earnings^*, this extraordinary statement

For the full year, net income surged to $5.71 per share from $3.89 per share in 2004. Annual revenue grew to $371 billion from $298.04 billion.

To put that into perspective, Exxon’s revenue for the year exceeded Saudi Arabia’s estimated 2005 gross domestic product of $340.5 billion, according to statistics maintained by the Central Intelligence Agency.

Now, I’m not talking about the statement about Exxon’s revenues, which is extraordinary, but about the helpful “putting into perspective” that the revenues were about the same as the GDP of Saudi Arabia.

Does the average reader know more — or have more perspective on that number — knowing that it’s comparable to the GDP of a country whose economics are not terribly familiar? Please! That’s about as helpful as saying the earnings in dollars is about the same as the number of miles in a light-week, or the number of sperm the average man produces in a year (about 390 billion). Even saying that it’s nearly 5% of the US national debt is more revealing. (It is, however, only about 50% greater than Wal*Mart’s yearly revenues.)

How about something that really gives a sense of how big such a number is. Something like: imagine that you were able to spend $10,000/hour, 24/7, 365.25 days a year, a pretty breathtaking pace — for perspective, that’s over 4 new Hummer H2s each day (use the surplus to buy the gas) . It would take you over 42 thousand years to spend Exxon’s yearly revenue.

Or, suppose we were to use the money to pay out to people an amount equal to the median US income in 2004: $44.4 thousand. Exxon’s yearly revenues would pay the median income to 8.3 million workers. For perspective, that’s about 11 workers for every mile between Earth and the Sun.

To my mind, either of these comparisons gives quite a bit more “perspective” than the GDP of Saudia Arabia
———-
^* Steve Quinn, “Exxon Mobil Posts Record Profit for 2005“, Associated Press / Yahoo! News, 30 January 2006.

Illegal but Very Cheap

Here’s another for the innumeracy files.
I heard on the radio today news that the Feds had “slapped” Wal-Mart with a fine of $11 Million to settle claims that Wal-Mart had illegally hired undocumented aliens. The spokesman for the Feds was deliriously happy with this result, evidently because it was the largest fine in the history of such things, and would, therefore, show Wal-Mart a thing or two.
Alas, he doesn’t understand the magnitudes of numbers. Wal-Mart’s yearly revenues are in the neighborhood of $160 Billion, a number over 14 thousand times larger than $11 Million. Thus, this fine is only o.oo7% of Wal-Mart’s yearly revenues. Even supposing that their profit margin is only 5%, this fine would still only be a miniscule 0.14% of their yearly profit.
Now, I’m not going to wade into the Wal-Mart good / Wal-Mart evil controversy, but I think most people should be able to see that a fine of 0.007% (that is, 1,000 times smaller than a typical sales tax), is pretty far from a serious punative fine. In fact, I’d say it’s a pretty cheap price to pay for doing business any way one wants.